Three-Act Tasks and a Neurodevelopmental Mystery

Three-Act Tasks. Notice and Wonder. Estimation 180. Math Talk. We learn about these leading practices for mathematics education in publications, on social media, and during professional learning events. Many educators find that these strategies are transforming today’s classrooms through increased engagement, constructive risk-taking, and rigorous problem-solving. We may be curious: From a cognitive development viewpoint, why are these techniques so effective?

Some recent work has given me new clues to solving this mystery. Over the past few years, I have become acquainted with the Math for All project that helps classroom and special education teachers view student strengths and challenges through a neurodevelopmental lens. Students who seem to struggle with math tasks may actually be struggling with a disconnection between their learning needs and classroom teaching strategies. Here is a simplified snapshot of the neurodevelopmental functions addressed in this project:

• Attention. Does the student stay focused on tasks, process incoming information, and regulate their responses?
• Language. Does the student understand the language in the problem? Can they read it? Can they explain their thinking?
• Higher-order Cognition. Does the student engage in complex and sophisticated thinking when required?
• Memory, Social Cognition, Motor Coordination, and Sequential and Spatial Ordering are among the other functions addressed by Math for All.

It occurs to me that leading mathematical practices can effectively support students with different strengths and challenges in neurodevelopmental areas. In this article, we will look at a high-quality three-act task implemented with these leading practices. We will see how students solve their mathematical mystery as their teacher solves the teaching mystery of reaching all students in the inclusive elementary classroom.

Three-Act Tasks. Three-act tasks are dramatized mathematics investigations structured in a three-part sequence and often presented through Web-based videos. Act one is a lesson launch, typically presenting a thought-provoking phenomenon for students to observe and ponder. Act two is a period of exploration in which students request clarifying data and then answer their own questions about what they observed in the first act. Act three brings the problem to a conclusion and offers students the opportunity to reflect on their solutions.

Getting Started with Array-bow of Colors, Act One. Array-Bow of Colors is a fourth-grade three-act task published by Graham Fletcher and freely available on the Web. (Links to all referenced resources are provided below.) In Act One, students collectively watch a fascinating, fast-motion video of a person emptying small packs of Skittles candy into a large jar. Students’ immersion in the video provides the first clues to our teaching mystery. Engaging students who have attention challenges can be more successful when the task commands their attention!

Additionally, sharing a phenomenon through video can increase access for students who have strengths in visual processing but have challenges with language, especially text.

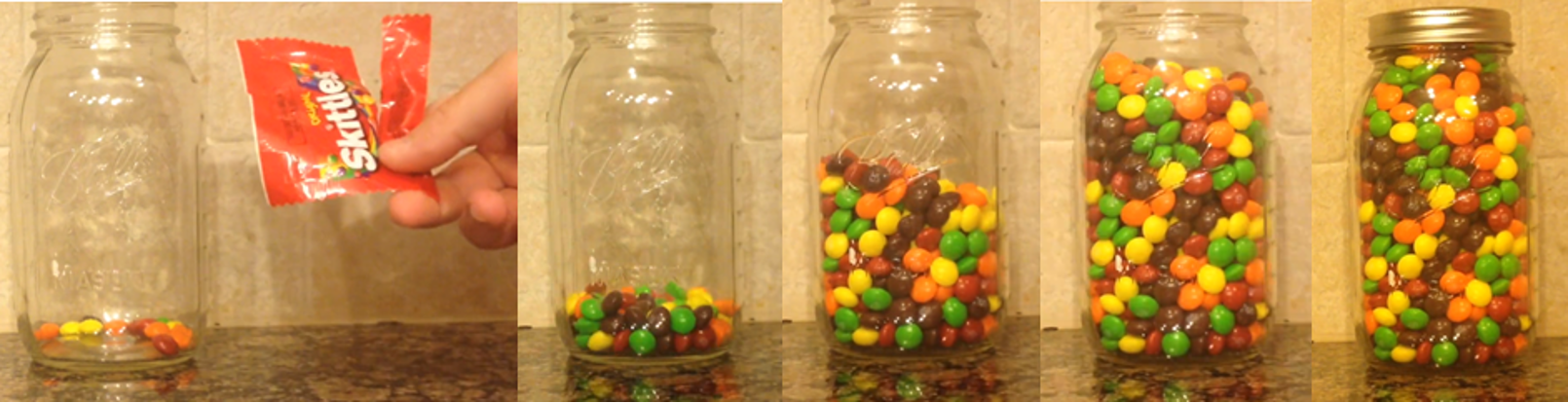
Notice and Wonder, Math Talk. This task is perfect for employing the “notice and wonder” strategy that is well-documented on the website of the National Council of Teachers of Mathematics (NCTM). The teacher poses questions to the class: “What do you notice? What do you wonder?” Students are encouraged to share their observations and engage their own curiosity to recognize intriguing problems.

Let us envision a student who has difficulty with social cognition. She states: “I noticed someone pouring Skittles into a jar.”

The teacher is versed in math talk, employing talk moves to orchestrate productive discourse, and decides to stay with this student and probe for more ideas. The teacher asks, “What else did you notice?” The student continues, “There were a lot of packages … so many Skittles in the jar!” And now we have a second clue: Intentional math talk can help students with strengths in language but who have social challenges by providing safe entry points into discourse.

The teacher asks the whole class what they notice and wonder. Students call upon their active working memory of the video. Through the use of partner talk and table talk, each student finds that their voice, and every voice, matters. Their collective wonder turns into a class mission:

We want to find out how many Skittles are in that jar!

This is student-centered learning. The students have effectively conceived their own mystery, designed their own mathematics problem. Now, they must solve it, one clue at a time!

Estimation 180. It is time to estimate, and Estimation 180 is a helpful approach for many rich math tasks. Students make three estimates, working in facilitated pairs or groups. The first estimate is reasonable yet intentionally low. The second is reasonable yet intentionally high. The third estimate represents the students’ best effort at predicting the result. A low estimate of five Skittles in the jar would not pass the “reasonable” test. A high estimate of one billion would simply be silly. So, the teacher asks the students to take responsibility and apply rigor to their estimates, ultimately recording the results for all to see.

Consider how neurodevelopmental functions are addressed through Estimation 180.

• Students can recall their estimates as they are now on the blackboard. Memory – supported.
• The three-estimate strategy gives them a framework to support thinking. Higher-order cognition – supported.
• With all students contributing to the group estimates, their social engagement is productive and safe. Social cognition – supported.
• There is excitement in the room. Which team made the best estimates? Attention – supported.
  

Act Two. Act Two poses the questions “How can we solve our mystery? What information will help us find out how many candies are in this jar?” As students ponder these questions, their conversation steers them toward the clues, the data, that they seek. The students decide that they would like to know how many Skittles are in one package and how many packages are in the jar.

As Act Two unfolds, students see pictures of the emptied Skittles packages and learn that there are 58 in total. They see a single package with its emptied contents on display: 14 Skittles. They now have two clues, two crucial data points: 58 packages and 14 Skittles per package. The mathematics is taking shape. As Sherlock Holmes would say, “The game is afoot!”